[연세대논술]2020학년도 연세대학교 수시모집 논술시험 오전

 

https://youtu.be/RF95YP4OYM0(구독과 좋아요!!)

 

[문제 1]

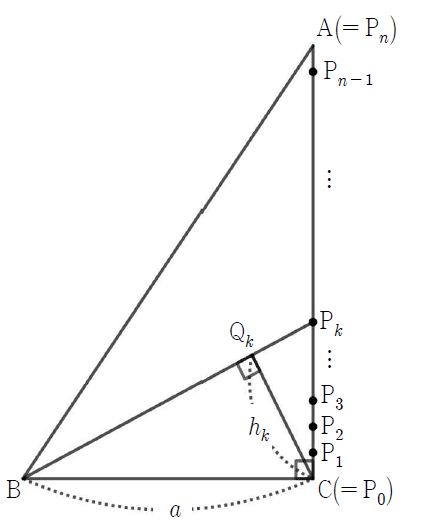
그림과 같이 $\displaystyle \mathrm { \overline {AC}} =1 $, $\displaystyle \overline {\mathrm { BC }} =a $ ($\displaystyle a>0 $)이고 $\displaystyle \mathrm { \angle BCA}= \frac {\pi } {2} $인 삼각형 $\displaystyle \mathrm { ABC }$가 있다. 자연수 $\displaystyle n $에 대하여 선분 $\displaystyle \mathrm { CA} $를 $\displaystyle n $등분한 각 분점을 점 $\displaystyle \mathrm { C }$에서 가까운 것부터 차례로 $\displaystyle \mathrm { P _ {0} ( =C) }$, $\displaystyle \mathrm { P _ {1} }$, $\displaystyle \mathrm { P _ {2} }$, $\displaystyle \mathrm { P _ {3} }$, $\displaystyle \cdots $, $\displaystyle \mathrm { P} _ { n-1 } $, $\displaystyle \mathrm { P} _ { n } ( = \mathrm { A}) $이라 하자. $\displaystyle 1 \leq k \leq n $인 자연수 $\displaystyle n $에 대하여 선분 $\displaystyle \mathrm { BP} _ { k } $에 내린 수선의 발을 $\displaystyle \mathrm { Q} _ { k } $라 하고, 선분 $\displaystyle \mathrm { CQ }_ { k } $의 길이를 $\displaystyle h _ {k} $라 하자. $\displaystyle h _ {k} $의 평균을 $\displaystyle H _ {n} $이라 할 때, $\displaystyle \lim\limits _ {n \rightarrow \infty } {H _ {n} } $을 구하시오. [10점]

 

 

 

 

 

 

[문제 2]

급수 $\displaystyle 1 ^ {2} +m \times 2 ^ {2} +3 ^ {2} +m \times 4 ^ {2} +5 ^ {2} +m \times 6 ^ {2} + \cdots $에서 첫째항부터 제$\displaystyle n $번째 항까지의 부분합을 $\displaystyle S _ {n} $라 할 때, $\displaystyle \lim\limits _ {n \rightarrow \infty } { \frac {S _ {n} } {n ^ {3} } } $이 자연수가 되도록 하는 자연수 $\displaystyle m $의 형태를 나타내시오. [10점]

 

 

 

 

[제시문]

좌표평면에서 벡터 $\displaystyle \overrightarrow {a} $에 대한 다음의 두 명제 $\displaystyle p _ {1} ,~p _ {2} $가 있다.

$\displaystyle p _ {1} ~:~ $$\displaystyle \overrightarrow {a} + \overrightarrow {b} = \overrightarrow {v} $와 $\displaystyle \left | \overrightarrow {v} \right | \leq \left | \overrightarrow {a} \right | + \left | \overrightarrow {b} \right | \leq 2 \left | \overrightarrow {v} \right | $를 만족시키는 벡터 $\displaystyle \overrightarrow {b} $가 존재한다.

$\displaystyle p _ {1} ~:~ $$\displaystyle \overrightarrow {a} + \overrightarrow {b} = \overrightarrow {v} $와 $\displaystyle \left | \overrightarrow {a} \right | =m $, $\displaystyle \left | \overrightarrow {b} \right | =n $을 만족시키는 벡터 $\displaystyle \overrightarrow {b} $가 존재한다. (단, $\displaystyle m $과 $\displaystyle n $은 $\displaystyle 0<m<n $인 상수이다.)

 

[문제 3-1] 벡터 $\displaystyle \overrightarrow {v} = \left ( c,~0 \right ) $일 때, 명제 $\displaystyle p _ {1} $을 만족시키는 위치벡터 $\displaystyle \overrightarrow {a} $의 종점이 이루는 도형을 $\displaystyle c $를 이용하여 나타내시오. (단, $\displaystyle c $는 양의 실수이다.) [10점]

 

[문제 3-2] 명제 $\displaystyle p _ {2} $를 만족시키는 벡터 $\displaystyle \overrightarrow {a} $의 집합을 $\displaystyle S $라고 할 때, 집합 $\displaystyle S $의 원소의 개수가 $\displaystyle 2 $가 되는 벡터 $\displaystyle \overrightarrow {v} $의 조건을 $\displaystyle m $과 $\displaystyle n $을 사용하여 나타내시오. [10점]

 

 

 

 

 

[제시문]

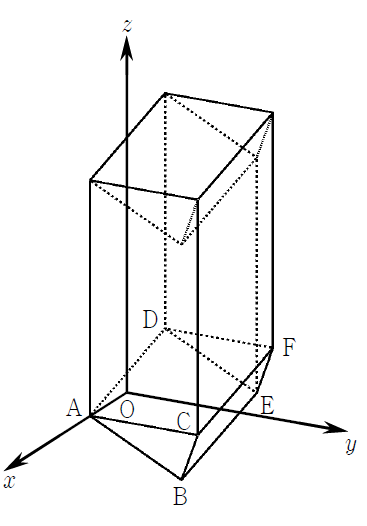
그림과 같이 좌표공간에서 $\displaystyle \mathrm { A} \left ( 1,~0,~0 \right ) $, $\displaystyle \mathrm { B} \left ( 0,~1,~-2 \right ) $, $\displaystyle \mathrm { C }\left ( 1,~2,~0 \right ) $, $\displaystyle \mathrm { D} \left ( -1,~0,~1 \right ) $, $\displaystyle \mathrm { E} \left ( -2,~1,~-1 \right ) $, $\displaystyle \mathrm { F} ( -1,~2,~1) $를 꼭짓점으로 하는 삼각기둥 $\displaystyle \mathrm { ABC-DEF} $를 $\displaystyle z $축의 방향으로 $\displaystyle 6 $만큼 평행이동하는 동안 삼각기둥 $\displaystyle \mathrm { ABC-DEF} $가 그리는 다면체를 $\displaystyle V $라 하자. 다음 물음에 답하시오.

 

[문제 4-1] 다면체 $\displaystyle V $와 평면 $\displaystyle z=3 $이 만나서 생기는 단면의 모양과 넓이를 구하시오. [10점]

[문제 4-2] 다면체 $\displaystyle V $의 부피를 구하시오. [10점]