-
[울산과고]2018년 경기과학고 1-1학기 중간고사[더플러스수학학원]과학고 2020. 5. 8. 15:59반응형
울산 옥동에 있는 울산과고전문 더플러스수학학원입니다. 예비울산과고1학년 학생들은 울산과고1학년 1학기 중간고사에 대해 많이 걱정과 기대를 가지고 지금 공부하고 있겠죠? 경기과고 내신기출문제를 가지고 학원에서 시험을 쳐봤습니다. 어여웠죠? 2월말까지의 여러분의 공부가 울산과고2년 또는 3년을 결정하겠죠! 열심히 합시다.
경기과고내신기출문제와 그 풀이와 풀이동영상입니다.
1. $\displaystyle x $에 대한 이차식 $\displaystyle x ^ {2} +ax+bc $와 $\displaystyle x ^ {2} +bx+ac $의 최대공약수가 $\displaystyle x $에 대한 일차식일 때, 다음 물음에 답하시오. (단, $\displaystyle abc \neq 0 $이다.) [총 $\displaystyle 8 $점]
(1) $\displaystyle x $에 관한 이차식 $\displaystyle x ^ {2} +ax+bc $와 $\displaystyle x ^ {2} +bx+ac $의 최소공배수를 구하고, 그 과정을 서술하시오. [$\displaystyle 4 $점]
(2) $\displaystyle \frac {\left ( a ^ {3} +b ^ {3} +c ^ {3} \right ) \left ( ab+bc+ca \right )} {a ^ {3} bc+ab ^ {3} c+abc ^ {3} } $의 값을 구하고, 그 과정을 서술하시오. [$\displaystyle 4 $점]
2. 다항식 $\displaystyle f \left ( x \right ) $를 $\displaystyle \left ( x ^ {2} +1 \right ) ^ {2} ,~ \left ( x ^ {2} +2 \right ) ^ {2} $으로 나누었을 때의 나머지가 각각 $\displaystyle x ^ {3} -2x ^ {2} +3x+1,~-x ^ {3} -4x ^ {2} -2 $이다. 다항식 $\displaystyle f \left ( x \right ) $를 $\displaystyle \left ( x ^ {2} +1 \right ) \left ( x ^ {2} +2 \right ) $로 나누었을 때의 나머지를 구하고, 그 과정을 서술하시오. [$\displaystyle 7 $점]
3. $\displaystyle x,~y $에 관한 다항식 $\displaystyle x ^ {7} -y ^ {7} - \left ( x-y \right ) ^ {7} $을 $\displaystyle 3 $차 이하의 정수 계수 다항식으로 인수분해하고, 그 과정을 서술하시오. [$\displaystyle 8 $점]
4. 방정식 $\displaystyle x ^ {6} -2x ^ {5} +x ^ {4} -7x ^ {3} +x ^ {2} -2x+1=0 $의 서로 다른 네 허근을 $\displaystyle \alpha _ {i} \left ( i=1,~2,~3,~4 \right ) $라고 하자. $\displaystyle \left ( 1+2 \alpha _ {1} \right ) \left ( 1+2 \alpha _ {2} \right ) \left ( 1+2 \alpha _ {3} \right ) \left ( 1+2 \alpha _ {4} \right ) $의 값을 구하고, 그 과정을 서술하시오. [$\displaystyle 6 $점]
5. 방정식 $\displaystyle 2x ^ {2} -y ^ {2} +xy-6x+3y-2=0 $을 만족시키는 양의 정수 $\displaystyle x,~y $의 순서쌍 $\displaystyle \left ( x,~y \right ) $를 모두 구하고, 그 과정을 서술하시오. [$\displaystyle 6 $점]
6. 양수 $\displaystyle x,~y,~z $에 대하여 다음 물음에 답하시오. [총 $\displaystyle 14 $점]
(1) $\displaystyle x ^ {2} +y ^ {2} +z ^ {2} =1 $을 만족시킬 때, $\displaystyle \frac {1} {x} + \frac {1} {y} + \frac {1} {z} $의 최솟값을 구하고, 그 과정을 서술하시오. [$\displaystyle 6 $점]
(2) $\displaystyle x ^ {2} +y ^ {2} +z ^ {2} =6,~xyz=2 $을 만족시킬 때, $\displaystyle z $의 최댓값을 구하고, 그 과정을 서술하시오. [$\displaystyle 8 $점]
7. 좌표평면 위의 직선 $\displaystyle ax+by+c=0 $과 임의의 점 $\displaystyle \mathrm { P} \left ( x _ {1} ,~y _ {1} \right ) $ 사이의 거리를 구하고, 그 과정을 서술하시오. (단, $\displaystyle ab \neq 0 $이다.) [$\displaystyle 6 $점]
8. 원 $\displaystyle x ^ {2} +y ^ {2} =16 $의 접선 $\displaystyle g $에 대하여 직선 $\displaystyle g $가 두 직선
$$\displaystyle l~:~3x+y-2=0 $$
$$\displaystyle l ^ { ' }~ :~x+3y-6=0 $$
과 만나는 점을 각각 $\displaystyle \mathrm { P,~Q }$라고 하고, 두 직선 $\displaystyle l,~l ^ { ' } $의 교점을 $\displaystyle \mathrm { R }$라고 하자. 삼각형 $\displaystyle \mathrm { PQR} $가 $\displaystyle \mathrm { \overline {PR} = \overline {QR} }$인 이등변삼각형이 되도록 하는 접선 $\displaystyle g $의 방정식을 구하고, 그 과정을 서술하시오. [$\displaystyle 6 $점]
9. $\displaystyle \mathrm { \overline {AB} }=a,~ \mathrm { \overline {BC}} =b $인 직사각형 $\displaystyle \mathrm { ABCD }$에 대하여 삼각형 $\displaystyle \mathrm { ACD }$의 내부의 점 $\displaystyle \mathrm { P }$에서 변 $\displaystyle \mathrm { AB,~AD }$에 내린 수선의 발을 각각 $\displaystyle \mathrm { E,~F} $라고 하고 $\displaystyle \mathrm { \overline {PE} }=n,~ \mathrm { \overline {PF} }=m $이라고 하자. 또, 점 $\displaystyle \mathrm { E} $를 중심으로 하고 반지름의 길이가 $\displaystyle n $인 원과 선분 $\displaystyle \mathrm { EB }$의 교점을 $\displaystyle \mathrm { G }$라고 하자.
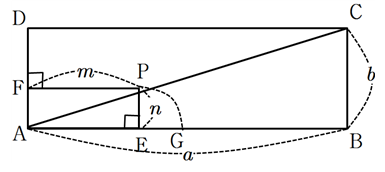
점 $\displaystyle \mathrm { B }$가 선분 $\displaystyle \mathrm { AG} $를 $\displaystyle m~:~n $으로 외분할 때, 다음 식의 참, 거짓을 판단하고 그 이유를 서술하시오. (단, $\displaystyle m>n $이다.)
(1) $\displaystyle \frac {n} {m} < \frac {b-n} {a-m} $ [$\displaystyle 3 $점]
(2) $\displaystyle \frac {2} {\overline {\mathrm { AG } }} = \frac {1} {\overline {\mathrm { AE } }} + \frac {1} {\overline {\mathrm { AB }} } $ [$\displaystyle 3 $점]
10. 좌표평면 위의 두 점 $\displaystyle \mathrm { P }\left ( a,~b \right ) ,~ \mathrm { Q} \left ( c,~d \right ) $가 직선 $\displaystyle y=kx-1 $에 대하여 서로 대칭이다. 다음 물음에 답하시오. (단, $\displaystyle k $는 상수이다.) [총$\displaystyle 12 $점]
(1) $\displaystyle k=1 $일 때, 점 $\displaystyle \mathrm { Q} $의 좌표를 $\displaystyle a,~b $에 관한 식으로 나타내고, 그 과정을 서술하시오. [$\displaystyle 3 $점]
(2) 점 $\displaystyle \mathrm { P }$가 포물선 $\displaystyle C~ :~y=x ^ {2} $위를 움직일 때, 점 $\displaystyle \mathrm { Q }$의 자취가 포물선 $\displaystyle C $와 만나지 않기 위한 실수 $\displaystyle k $의 범위를 구하고, 그 과정을 서술하시오. [$\displaystyle 4 $점]
(3) 점 $\displaystyle \mathrm { P} \left ( -1,~2 \right ) $에 대하여 실수 $\displaystyle k $가 변할 때, 점 $\displaystyle \mathrm { Q} $의 자취의 방정식을 구하고, 그 과정을 서술하시오. [$\displaystyle 5 $점]
11. 다음 세 부등식 $\displaystyle P,~Q,~R $
$\displaystyle P~:~y \geq 0 $
$\displaystyle Q~:~ \sqrt {3} x+y-3 \leq 0 $
$\displaystyle R~:~x- \sqrt {3} y+3 \sqrt {3} \geq 0 $
을 동시에 만족시키는 영역을 $\displaystyle T $라고 하고, $\displaystyle T $에 속하는 임의의 점에서 세 부등식 $\displaystyle P,~Q,~R $의 영역의 경계선에 내린 수선의 길이를 각각 $\displaystyle p,~q,~r $라고 하자. $\displaystyle p ^ {2} -q ^ {2} -r ^ {2} =0 $일 때, $\displaystyle p $의 최댓값을 구하고, 그 과정을 서술하시오.
12. 포물선 $\displaystyle C~:~x ^ {2} =4y $위의 점 $\displaystyle P $에서의 접선을 $\displaystyle l _ {1} $, 점 $\displaystyle \mathrm { P} $를 지나고 직선 $\displaystyle l _ {1} $에 수직인 직선을 $\displaystyle g _ {1} $이라고 하고, 포물선 $\displaystyle C $위의 점 $\displaystyle \mathrm { Q} $에서의 접선을 $\displaystyle l _ {2} $, 점 $\displaystyle \mathrm { Q }$를 지나고 직선 $\displaystyle l _ {2} $에 수직인 직선을 $\displaystyle g _ {2} $라고 하자. 두 직선 $\displaystyle l _ {1} ,~l _ {2} $의 교점을 $\displaystyle \mathrm { R }$, 두 직선 $\displaystyle g _ {1} ,~g _ {2} $의 교점을 $\displaystyle \mathrm { S} $라고 하자. 두 직선 $\displaystyle l _ {1} ,~l _ {2} $가 서로 수직이 되도록 두 점 $\displaystyle \mathrm { P,~Q }$가 포물선 $\displaystyle C $ 위를 움직일 때, 다음 물음에 답하시오. [총 $\displaystyle 14 $점]
(1) 점 $\displaystyle \mathrm { R} $의 자취의 방정식을 구하고, 그 과정을 서술하시오. [$\displaystyle 4 $점]
(2) 점 $\displaystyle \mathrm { S }$의 자취의 방정식을 구하고, 그 과정을 서술하시오. [$\displaystyle 4 $점]
(3) 선분 $\displaystyle \mathrm { RS }$를 지름으로 하는 원의 넓이의 최솟값을 구하고, 그 과정을 서술하시오. [$\displaystyle 6 $점]
울산과고내신전문 더플러스수학학원을 찾아오시려면
반응형'과학고' 카테고리의 다른 글
[더플러스수학]2019년 울산과고2 심화수학 기출 20번 -gambler's ruin (0) 2020.06.24 2020년 과고2 확률과 통계 - 비둘기집 원리 프린트 (0) 2020.06.16 2019학년도 상산고 1학기 중간고사 및 풀이 (0) 2020.04.25 과고1학기 중간고사대비 심화문제 모음(2) (0) 2020.04.02 과학고 2020년도 1-1 중간고사대비(18회) (0) 2020.03.14